Circular Measure (part 2)

Circular Measure (Part 2)

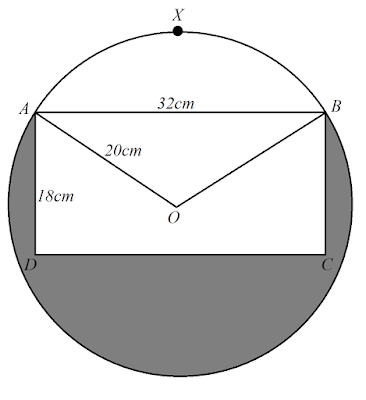
1. The diagram shows a metal plate $ABCDEF$ which has been made by removing the two shaded regions from a circle of radius $10cm$ and centre $O$. The parallel edges $AB$ and $ED$ are both of length $12cm$.

     i) Show that the angle $DOE$ is $1.287$ radians, correct to $4$ significant figures.

     ii) Find the perimeter of the metal plate

     iii) Find the area of metal plate

2. The diagram shows two circles, $C_1$ and $C_2$, touching at the point $T$. Circle $C_1$ has centre $P$ and radius $8cm$; circle $C_2$ has centre $Q$ and radius $2cm$. Points $R$ and $S$ lie on $C_1$ and $C_2$ respectively, and $RS$ is a tangent to both circles.

     i) Show that $RS=8cm$

     ii) Find angle $RPQ$ in radians correct to $4$ significant figures

     iii) Find the area of the shaded region

3. The diagram shows points $A$, $C$, $B$, $P$ on the circumference of a circle with centre $O$ and radius $3cm$. Angle $AOC$ = angle $BOC$= $2.3$ radians

     i) Find angle $AOB$ in radians, correct to $4$ significant figures.

     ii) Find the area of the shaded region $ACBP$, correct to $3$ significant figures.

4. The diagram shows a rhombus $ABCD$. Points $P$ and $Q$ lie on the diagonal $AC$ such that $BPD$ is an arc of a circle with centre $C$ and $BQD$ is an arc of a circle with centre $A$. Each side of the rhombus has length $5cm$ and angle $BAD=1.2$ radians

     i) Find the area of the shaded region $BPDQ$

     ii) Find the length of $PQ$

5. In the diagram, $OAB$ is an isosceles triangle with $OA=OB$ and angle $AOB=2\theta$ radians. Arc $PST$ has centre $O$ and radius $r$, and the line $ASB$ is a tangent to the arc $PST$ at $S$.

     i) Find the total area of the shaded regions in term of $r$ and $\theta$

     ii) In the case where $\theta=\frac{1}{3}\pi$ and $r=6$, find the total perimeter of the shaded regions, leaving your answer in terms of $\sqrt{3}$ and $\pi$.

6. (a) A circle is divided into 6 sectors in such a way that the angles of the sectors are in arithmetic progression. The angle of the largest sector is $4$ times the angle of the smallest sector. Given that the radius of the circle is $5cm$, find the perimeter of the smallest sector.

(b) The first, second and third terms of a geometric progression are $2k+3$, $k+6$ and $k$, respectively. Given that all the terms of the geometric progression are positive, calculate

     i) the value of the constant $k$

     ii) the sum to infinity of the progression

7. In the diagram, $AB$ is an arc of a circle, centre $O$ and radius $6cm$, and angle $AOB=\frac{1}{3}\pi$ radians. The line $AX$ is a tangent to the circle at $A$, and $OBX$ is a straight line.

     i) Show that the exact length of $AX$ is $6\sqrt{3}cm$

     Find, in terms of $\pi$ and $\sqrt{3}$,

     ii) the area of the shaded region

     iii) the perimeter of the shaded region

8. The diagram represents a metal plate $OABC$, consisting of a sector $OAB$ of a circle with centre $O$ and radius $r$, together with a triangle $OCB$ which is right-angled at $C$. Angle $AOB=\theta$ radians and $OC$ is perpendicular to $OA$

     i) Find an expression in terms of $r$ and $\theta$ for the perimeter of the plate

     ii) For the case where $r=10$ and $\theta=\frac{1}{5}\pi$, find the area of the plate

9. The diagram shows a circle $C_1$ touching a circle $C_2$ at a point $X$. Circle $C_1$ has centre $A$ and radius $6cm$, and circle $C_2$ has centre $B$ and radius $10cm$. Points $D$ and $E$ lie on $C_1$ and $C_2$ respectively and $DE$ is parallel to $AB$. Angle $DAX=\frac{1}{3}\pi$ radians and angle $EBX=\theta$ radians.

     i) By considering the perpendicular distances of $D$ and $E$ from $AB$, show that the exact value of $\theta \sin^{-1}(\frac{3\sqrt{3}}{10})$.

     ii) Find the perimeter of the shaded region, correct to $4$ significant figures.

10. In the diagram, $ABCD$ is a parallelogram with $AB=BD=DC=10cm$ and angle $ABD=0.8$ radians. $APD$ and $BQC$ are arcs of circles with centre $B$ and $D$ respectively.

     i) Find the area of the parallelogram $ABCD$

     ii) Find the area of the complete figure $ABQCDP$

     iii) Find the perimeter of the complete figure $ABQCDP$

11. In the diagram, $ABC$ is an equilateral triangle of side $2cm$. the mid-point of $BC$ is $Q$. An arc of a circle with centre $A$ touches $BC$ at $Q$, and meets $AB$ at $P$ and $AC$ at $R$. Find the total area of the shaded regions, giving your answer in terms of $\pi$ and $\sqrt{3}$.

12. The diagram shows a metal plate made by removing a segment from a circle with centre $O$ and radius $8cm$. The line $AB$ is a chord of the circle and angle $AOB=2.4$ radians. Find

     i) The length of $AB$

     ii) The perimeter of the plate

     iii) The area of the plate

13. In the diagram, $AB$ is an arc of a circle with centre $O$ and radius $r$. The line $XB$ is a tangent to the circle at $B$ and $A$ is the mid-point of $OX$

     i) Show that angle $AOB=\frac{1}{3}\pi$ radians.

     Express each of the following in terms of $r$, $\pi$ and $\sqrt{3}$:

     ii) the perimeter of the shaded region

     iii) the area of the shaded region

14. The diagram shows a sector $OAB$ of a circle with centre $O$ and radius $r$. Angle $AOB$ is $\theta$ radians. The point $C$ on $OA$ is such that $BC$ is perpendicular to $OA$. The point $D$ is on $BC$ and the circular arc $AD$ has centre $C$.

     i) Find $AC$ in terms of $r$ and $\theta$

     ii) Find the perimeter of the shaded region $ABD$ when $\theta=\frac{1}{3}\pi$ and $r=4$, giving your answer as an exact value.